

Write up 7 Tangent Circles- BRENT ROBERTSON-
First of all this was a fantastic experience to work with Dr. Wilson on this sketch. Dr. Wilson, you are truly amazing and I am definitely amazed by the possibilities of the Construction of Circles Tangent to two given circles. Dr. Wilson helped me with this one because what I was doing was"drawing" with the Geometer's Sketchpad instead of actually constructing. What occurs when you draw rather than construct is your mathematical ideas won't stick together when you move points, lines and planes around; in other words, the wheels start to fall off of the train.
What we want to construct and investigate in this problem set is a circle that is Tangent to two other circles. The construction can be accessed at this sketch page: Tan circles 1 This Sketch has a script tool that will enable you to start with two green circles-one large and one small- with he large one enveloping the small one. We construct a red circle that is tangent to both green circles using ingenious means. Using the compass tool we can construct another circle with the same radius as circle C ( the small green one), with H as a center on circle A, the large green circle. One case would be that the center of our desired tangent circle is on the line conatiaining the center and point of tangency to circle A. This is because the centers of the tangent circles will go through the point of tangency. So our desired center is somewhere on line HA. To find that point we use the fact that the citer will be equidistant from C and L because that rdial distance will be the distlance of the tangent circle and the little green circle L. Since it lies on line HA we can find the perpendicular bisector of CL and find where it intersects HA. This is the center of one of our desired circles. There is another but let's enjoy this one for now. Try tracing the center of the red tangent circle and find ourt what you get as we animate the point of tangency H around the big green circle.
Here's what you should see:
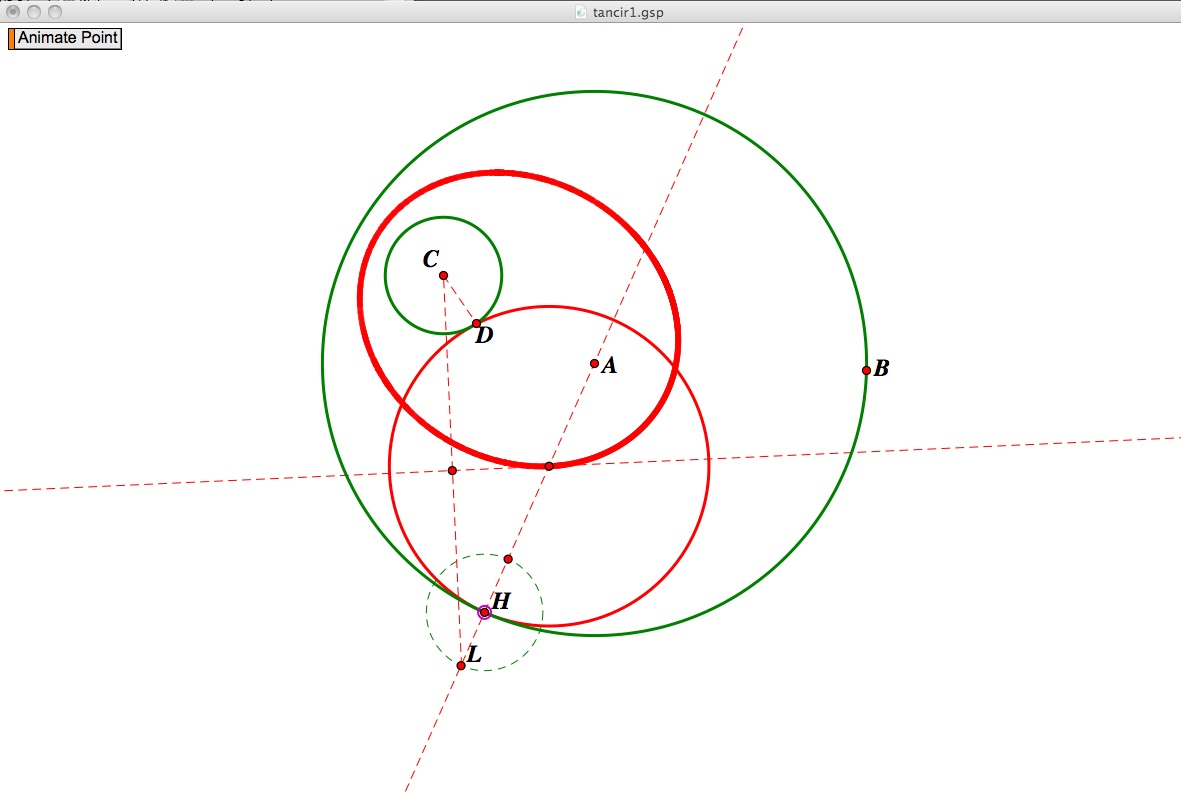
We can conjecture that the trace looks like an ellipse and it is in fact very easy to prove by the very definition of an ellipse. A and C are the foci (the centers of our original green circles) and the ellipse is traced by the constant sum of the distances of A and C from the foci. By definition the figure created is an ellips since it is the sum of the distances from the two centers of the tangent circles. There are also many more intersesting mathematical discoveries that can be discovered. What if the trace objects were the tangent lines and not the locus of equidistant sums?
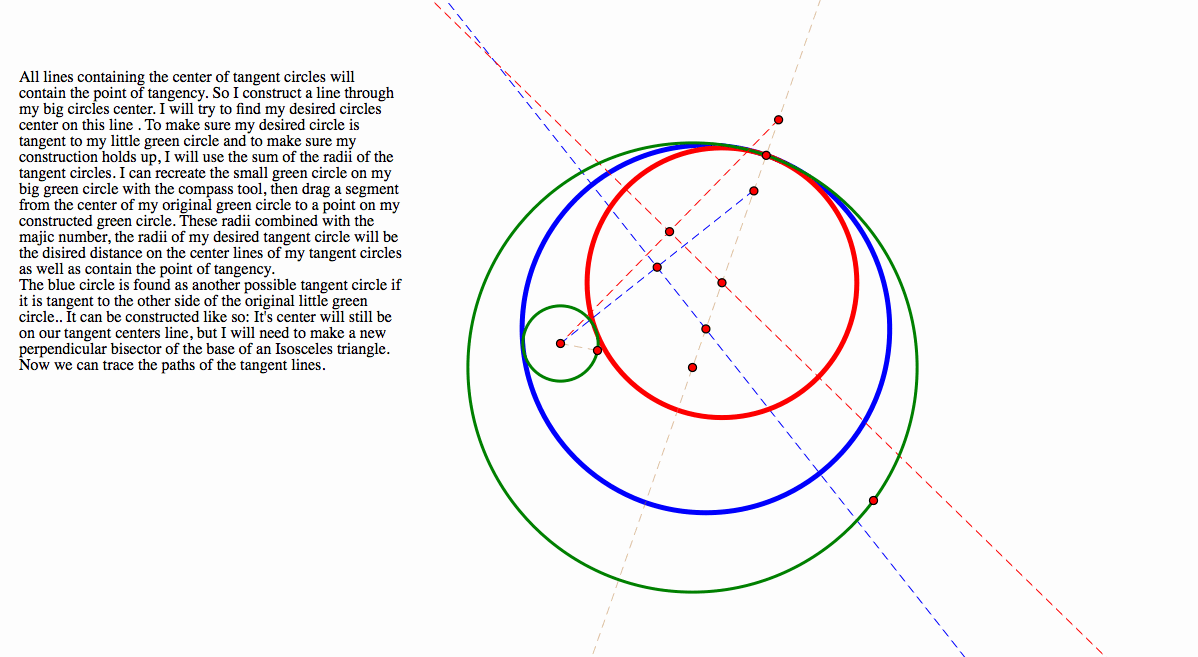
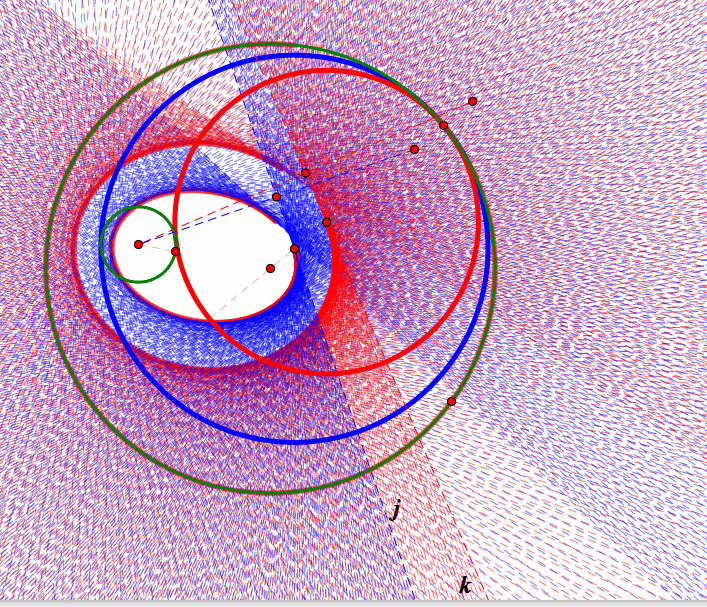
This give another lens to view the locus of points. Now this colorful drawing represents the envelope of the tangent lines. This envelop defines the locus more for what it is not than for what it is.